1. Tính giá trị của biểu thức A= căn bậc hai của x + 1/ căn bậc hai của x - 2 với x = 7 + 4 căn bậc hai của 3 2. Cho biểu thức B = căn bậc hai của x/ căn bậc hai của x + 1 + 1 - căn bậc
1. Tính giá trị của biểu thức 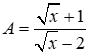 với
với ![]()
2. Cho biểu thức 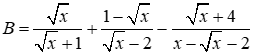 với
với ![]()
Chứng minh rằng ![]()
3. Tìm x để = ![]()
